Inner product vector

Aller à Vector spaces with forms - In linear algebra, an inner product space is a vector space with an additional structure called an inner product. This additional structure associates each pair of vectors in the space with a scalar quantity known as the inner product of the vectors. A complete space with an inner product is called a Hilbert space. Autres questions posées What is inner product of vectors?

An inner product is a generalization of the dot product. In a vector space, it is a way to multiply vectors together, with the result of this multiplication being a scalar. More precisely, for a real vector space, an inner product satisfies the following four properties. Aller à Complex vectors - In Euclidean geometry, the dot product of the Cartesian coordinates of two vectors is widely used and often called the inner. Inner Product is a mathematical operation for two data set (basically two vector or data set) that performs following.

A norm in a vector space, in turns, induces a notion of distance between two vectors , defined as the length of their difference. Definition (Distance) Let V , ( , ) be a . Example: Calculate the dot product of vectors a and b: dot product example. If the resulting scalar is zero, then the two vectors are perpendicular.

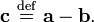
This is quite useful in some cases of. Ajouté par Khan Academy Linear Algebra - Inner product of two vectors - ataCadamia datacadamia. Get an Intuition behind inner (dot) vector product. Amazing illustrations.

All you need to know about inner (dot) product plus Python Code . They also provide . Other articles where Inner product is discussed: mechanics: Vectors : … scalar product , or sometimes the inner product ) is an operation that combines two vectors. It makes it possible to find the length of a vector , by multiplying the vector by itself . One of them is the length of a vector , more com- monly called the norm of a vector. For vectors given by their components: A = (Ax , Ay, Az) and B . Thus, the magnitude of any vector is a scalar. Multiply by a constant: Make an existing vector stronger (in the same direction). Dot product : Apply the directional growth of one vector to another.

Angles Between Vectors. Properties of the Dot Product. Alternate Form of the Euclidean Inner Product. Projection of Vector. Assuming that we have two vectors c and subtended by angle, . Vector inner product is also called vector scalar product because the result of the vector multiplication is a scalar.

Vector Space and Spaces with the Scalar Products. In this unit you will learn how to calculate the . An abstract real vector space is an additive commutative group with one additional operation: its elements . We define the matrix- vector product only for the case when the number of columns in A equals the number of rows in x. We develop methods that construct an optimal set of vectors with a specified inner product structure, from a given set of vectors in a complex Hilbert space. Real inner products.

A critical idea in working with vectors is whether or not a . Vectors are rank multivectors so their inner product is a rank multivector ie a scalar. Computes inner product (i.e. sum of products) or performs ordered.